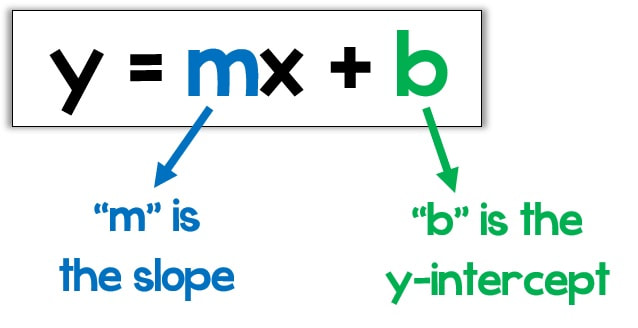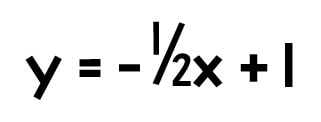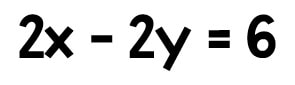Objectives:
- I can draw the graph of an equation in slope-intercept form
- I can write an equation in slope-intercept form from a given linear graph
- Given a linear equation, I can convert it into slope-intercept form
What is slope-intercept form?
Slope-intercept form is one way to write the equation of a linear line. We call it slope-intercept form because it is organized as that we can easily locate the slope and y-intercept of the line. For example, the general equation for slope-intercept form is:
Slope-intercept form is one way to write the equation of a linear line. We call it slope-intercept form because it is organized as that we can easily locate the slope and y-intercept of the line. For example, the general equation for slope-intercept form is:
In this form, we can easily spot the slope, m, and the y-intercept, b.
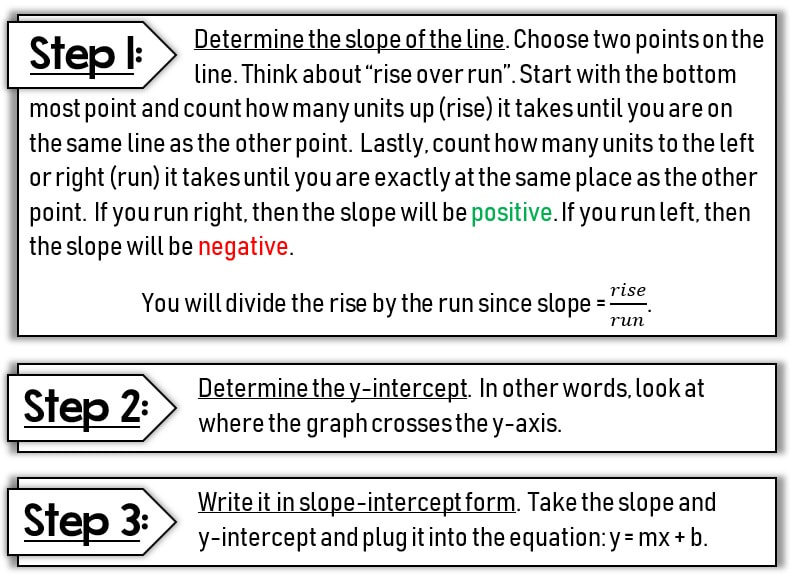
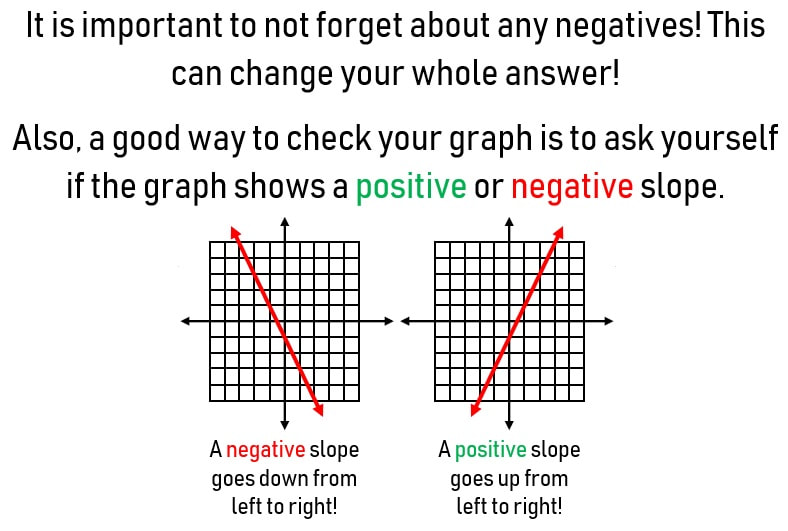
SLOPE: You have learned previously that the slope is the steepness of a line. You have heard the phrase "rise over run" many times now, also known as the change in y over the change in x.
Y-INTERCEPT: The y-intercept is the value of "y" when the line intercepts the y-axis.
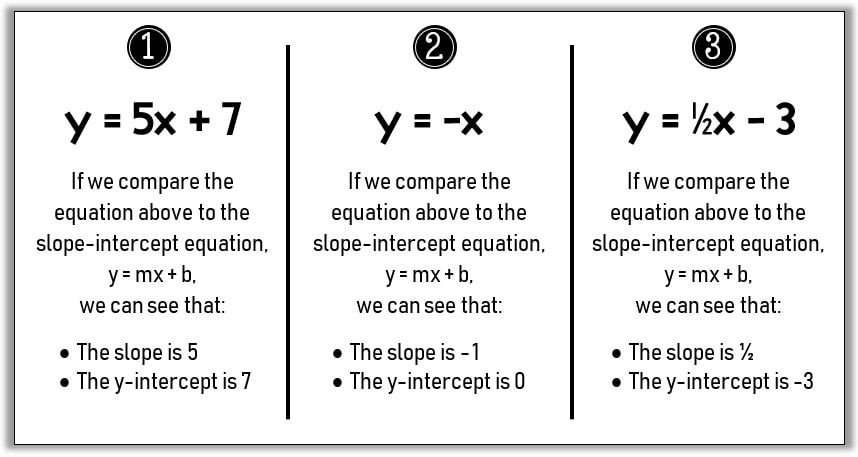
Now, let's look at some examples!
SLOPE: You have learned previously that the slope is the steepness of a line. You have heard the phrase "rise over run" many times now, also known as the change in y over the change in x.
Y-INTERCEPT: The y-intercept is the value of "y" when the line intercepts the y-axis.
Now, let's look at some examples!
Example #2 from above is usually a tricky equation! As seen, the slope is -1 because there is an "invisible" 1 in front of the x variable. In addition, there is no constant that is being added or subtracted. This means that the y-intercept is 0. We DO NOT say that there is no y-intercept. This would be incorrect because the graph has a y-intercept at 0. This means that the graph goes through the origin at (0,0).
Practice #1
Identify the slope and y-intercept of the following equation:
Click HERE to check your answer.
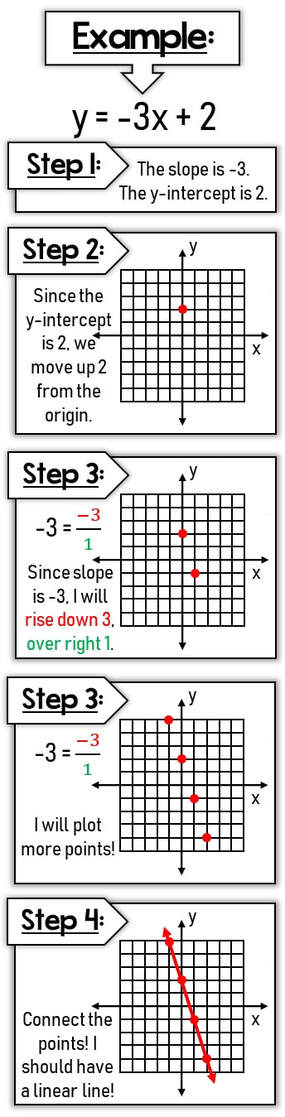
Graphing an Equation in Slope-Intercept Form
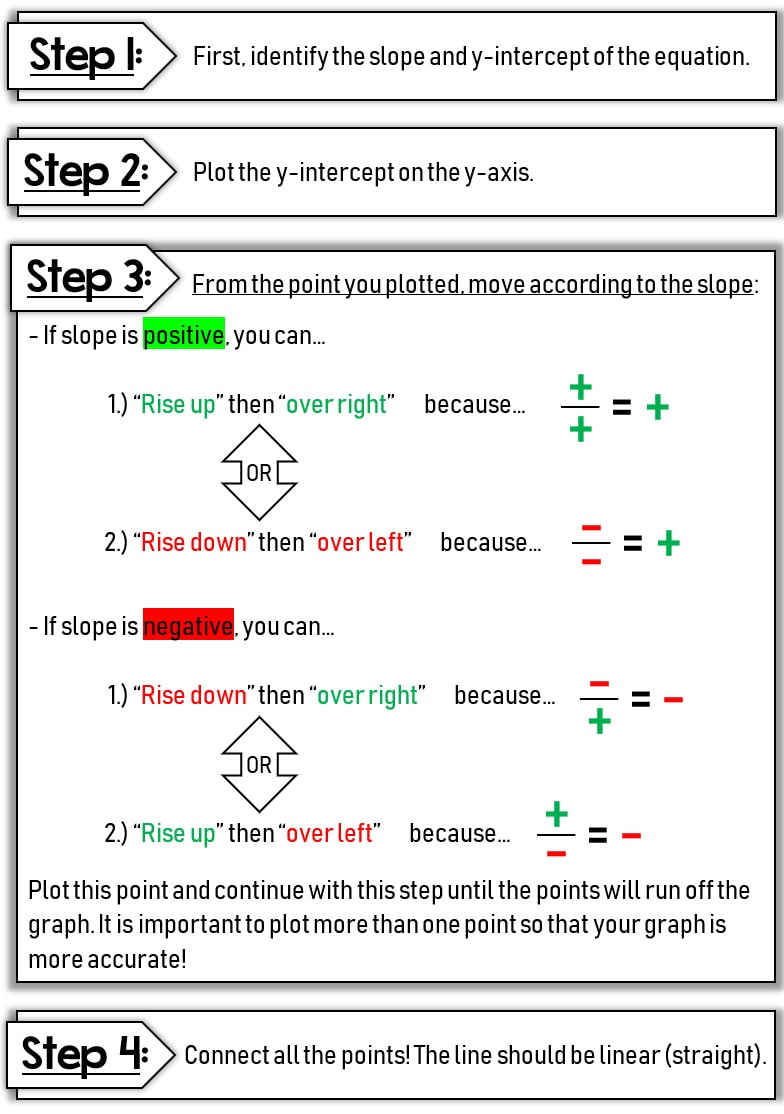
Given the same examples from above, we will graph the equations in slope-intercept form. As stated earlier, the "m" is the slope and the "b" is the y-intercept. This is all the information that we need to be able to graph. Let's look at the steps and an example:
Practice #2
Graph the following equation:
Click HERE to check your answer!
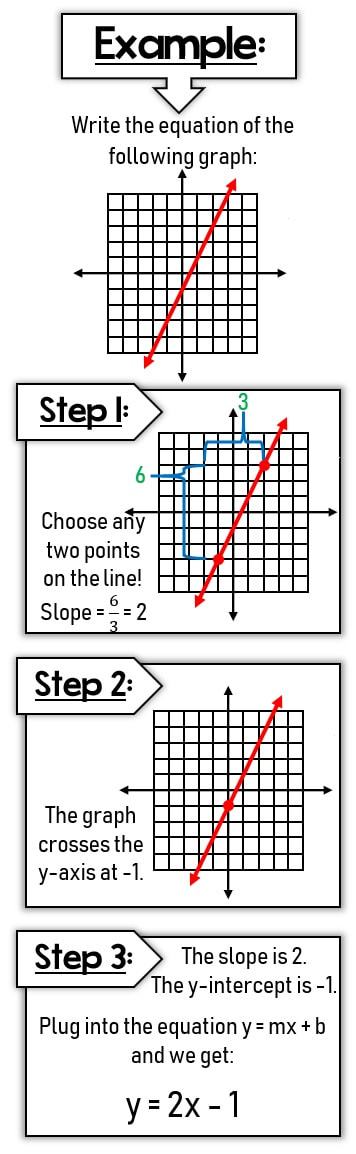
Writing an Equation in Slope-Intercept Form from a Linear Graph
You just learned how to graph an equation in slope-intercept form. Now, you are going to do the opposite by determining the slope-intercept form of a given linear graph. In order to write the linear equation of a graph, we need to know two things: (1) Where does the line cross the y-axis, and (2) What is the slope of the line. Let's look at the steps to follow and an example:
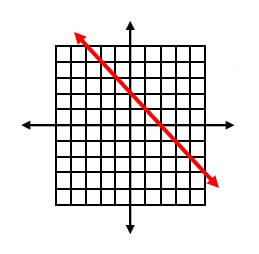
Practice #3
Determine the equation of the following graph:
Click HERE to check your answer!
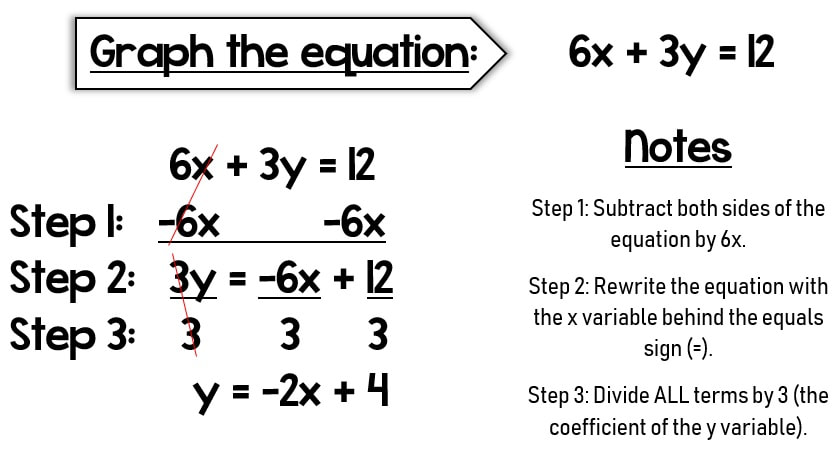
Converting into Slope-Intercept Form
Earlier, you learned how to graph an equation given in slope-intercept form. However, what if you are given a linear equation that is not in slope-intercept form? We have already discussed earlier that slope-intercept form makes it easier to recognize the slope and y-intercept. Hence, we will need to "convert" any linear equation into slope-intercept form. In other words, "solve for y"!
Let's look at an example:
Let's look at an example:
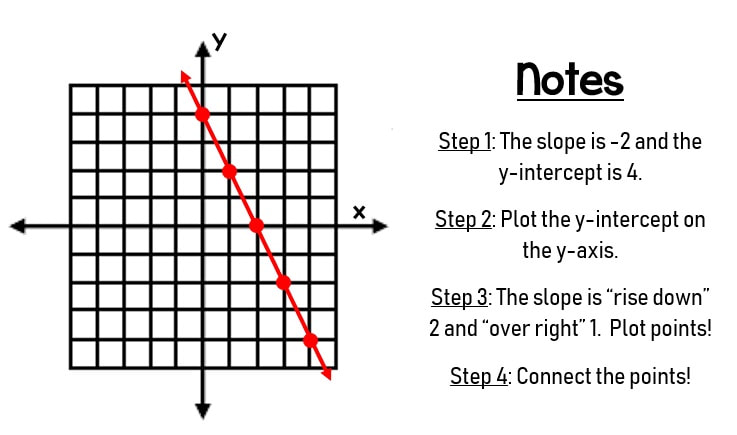
Now we can graph the equation since it is in slope-intercept form!
Practice #4
Graph the following equation:
Click HERE to check your answer!
Quiz
You will take the quiz after you have researched and taken notes on slope-intercept form.
CLICK HERE FOR QUIZ
CLICK HERE FOR QUIZ
(C) 2017 MATH IN DEMAND